
As in other emerging technological areas, significant research efforts are devoted for the miniaturization of electric devices. In this respect, modern concepts such as printable batteries and nanobatteries are gaining considerable attention. For these nanodevices, one technical issue which may be insignificant in usual applications cannot be neglected anymore: If the thickness of a conducting material is reduced to the sub-micrometer range, even small voltages (on the order of volts) may generate large electric fields which drive the material beyond linear response (established usually below few tens of kV/cm). In this nonlinear regime the scarcely investigated nonlinear effects start playing a significant role.
Although nonlinear effects in viscous liquids have recently gained a large interest in the glass community, their investigation for the case of conducting materials is scarce, with focus on ionic liquids and solid (glassy) ionic conductors. These studies reveal fascinating phenomena, not accessible via linear investigations and, to the best of our knowledge, such investigations are lacking for the scientifically and technologically highly relevant polymeric materials.
Specific to most polymers is that the conducting moieties are dynamically decoupled from the structural rearrangement of the embedding matrix. Although this decoupling phenomenon has been thoroughly investigated, the underlying mechanisms controlling its magnitude are still unresolved. Such large degrees of decoupling are technologically highly beneficial, as they reflect mechanical stability combined with high charge mobility. This “super-ionic” behavior, combined with the single-ion character of conductivity renders PolyILs promising candidates for the next generation of electrochemical applications.
Main Goal: To analyze the emerging and the impact of the nonlinear, high-field effects in ionic conducting polymer matrices
Our approach: The particular questions being addressed in this project are: To what extent does the nonlinear response of dynamically decoupled ionic materials differ from that of coupled systems such as ionic liquids? Can one always consider conducting materials subjected to large electrical fields as being in equilibrium? In other words, what is the timescale on which the nonlinear response of a given material reaches a steady state after a high field is applied or switched off? What microscopic mechanisms governed the amplitude of these effects?
The time evolution of the conductivity will be monitored in the so-called transient nonlinear experiments. The idea behind such experiments is sketched in Fig. 1(a). The procedure involves small-amplitude voltage cycles probing the linear response, followed by a step-like increase in the amplitude of the excitation for a time interval during which the material is driven out of equilibrium. By these means one monitors the time evolution of the nonlinear response at a given frequency. After a sufficiently long large-amplitude excitation the peak voltage is reduced so that the “dissipation” of the nonlinear effect can be monitored.
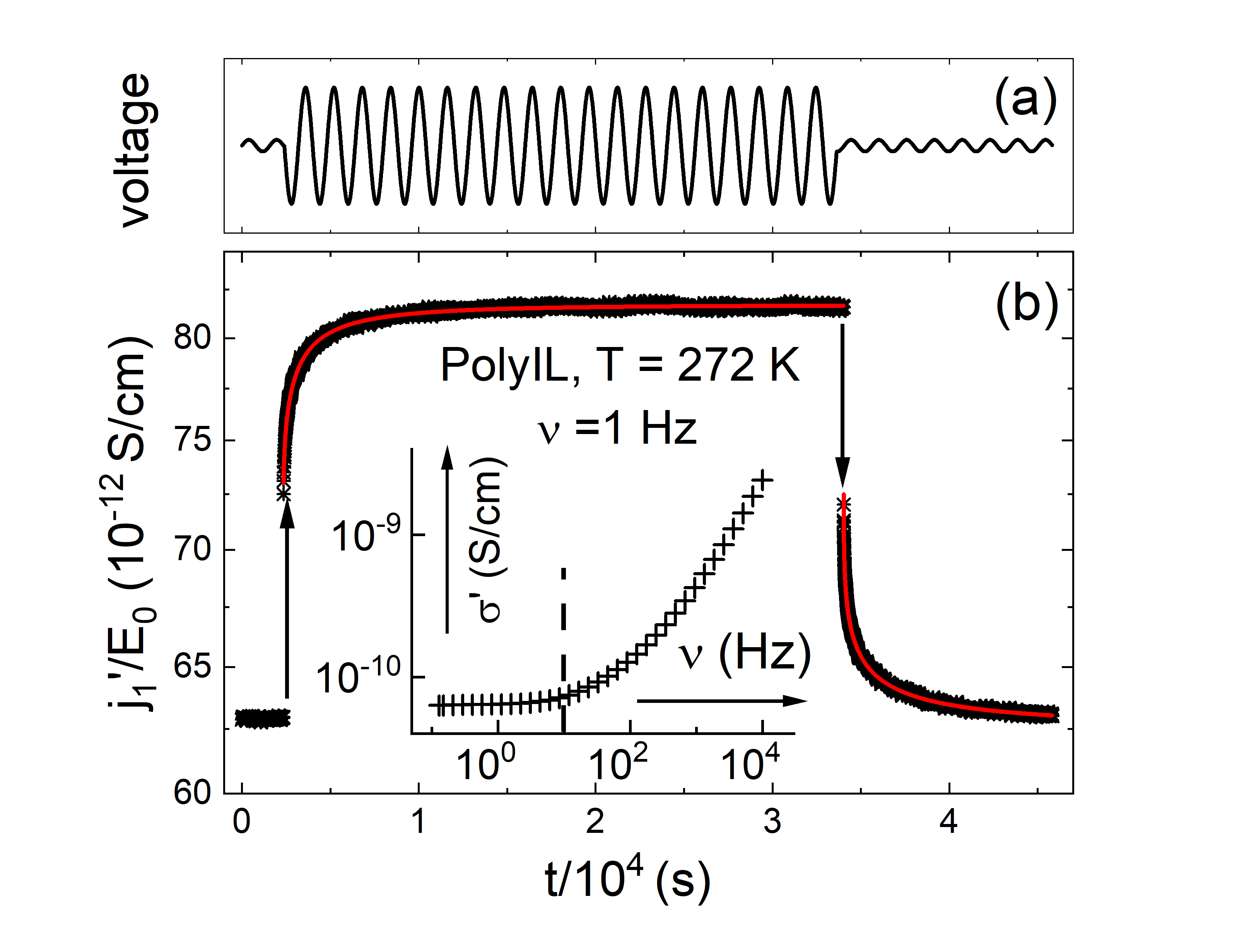
Fig. 1 (a) Schematic voltage profile used in the transient nonlinear investigations. (b) Time-resolved conductivity of a
PolyIL at 272 K, probed at 1 HZ with an AC field with E0 varying stepwise from 3 to 100 kV/cm and back to 3 kV/cm at
the times marked by the vertical arrows.
Brodin A., Gainaru C., Porokhonskyy V., Rössler E.A. “Evolution of dynamic susceptibility in molecular glass-formers – a critical assessment” J. Phys.: Cond. Matter 19, 205104 (2007)
Gainaru C., Böhmer R. “Coupling of the electrical conductivity to the structural relaxation, absence of physical aging on the time scale of the Debye process, and number of correlated molecules in the supercooled monohydroxy alcohol 2-ethylhexanol” J. Non-Cryst. Solids 356, 542 (2010)
Gainaru C., Böhmer R., Kahlau R., Rössler E.A. “Energy landscape in molecular glasses probed by high-resolution dielectric experiments” Phys. Rev. B 82, 104205 (2010)
Wieland F., Sokolov A.P., Böhmer R., Gainaru C. “Transient nonlinear response of dynamically decoupled ionic conductors” Phys. Rev. Lett. 121, 064503 (2018)